1. Start Chapter 4 Representing Precents.
We learned how to represent percents more than 100%.
We learned how to represent percents more than 100%.
We also learned how to represent percents less than 1 ex. 1/2%


What's the square root of 23?


HOMEWORK:
DUE TOMORROW!!

 Then We were told to find the perfect square.
Then We were told to find the perfect square. 
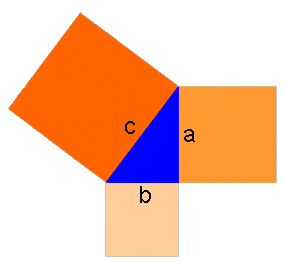 If A=15 cm2, B=5 cm2, then C would have to be 20 cm2. You see, in order for the triangle to be a right triangle the legs (two sides which are not the hypotenuse... the hypotenuse is the side opposite the right angle) area in A and B must equal the area of C. The Pythagorean Relationship states that A2 + B2 = C2.
If A=15 cm2, B=5 cm2, then C would have to be 20 cm2. You see, in order for the triangle to be a right triangle the legs (two sides which are not the hypotenuse... the hypotenuse is the side opposite the right angle) area in A and B must equal the area of C. The Pythagorean Relationship states that A2 + B2 = C2. 'If B is 25 cm2 and C is 90 cm2, What is A?' The thing people did wrong was that they added 25 and 90, HOWEVER, it said what is A! A isn't the hypotenuse therefore, you don't add you, subtract so... C-B=A = 90-25=65 cm2 So A was 65 cm2!
'If B is 25 cm2 and C is 90 cm2, What is A?' The thing people did wrong was that they added 25 and 90, HOWEVER, it said what is A! A isn't the hypotenuse therefore, you don't add you, subtract so... C-B=A = 90-25=65 cm2 So A was 65 cm2!



128-462 The first thing you would do is subtract 128 from 462. That leaves you with 334. You then divide 334 by 2. Once you do that you get 167. You then add 167 and 128 together to get a total of 295 which would be the number inbetween 128 and 462. I think we did two quick races.
The definition of:
At the end of class we were assigned page 85 numbers 5-12.
By the way...everybody should finish voting on the poll for the ELA blog. You only have 4 hours left!!
